ICTS

ICTS Exhibits at Sci560, Science Gallery Bengaluru

As part of the Sci560 exhibition organized by Science Gallery Bengaluru, the International Centre for Theoretical Sciences (ICTS) presents three unique exhibits that bridge the gap between cutting-edge scientific research and interactive public engagement. These exhibits showcase the synergy between theoretical physics, experimental analogies, and natural phenomena, inviting visitors to explore complex concepts through visually compelling and hands-on experiences.

The first exhibit draws an analogy between shallow-water waves and the merging of binary black holes, providing an accessible demonstration of gravitational wave generation. Using a ripple tank setup, this exhibit mirrors the gravitational waves produced during the inspiral and collision phases of black hole mergers, translating an otherwise abstract concept into a tangible, visual experience.

The second exhibit, developed in collaboration with NCBS, uses slime mold to model Bengaluru's metro network. The experiment explores how nature’s problem-solving abilities can inspire the design of efficient urban transit systems. Visitors can observe how slime mold forms networks between food sources placed on a map of Bengaluru, mimicking the optimal layout for metro lines and offering insights into biologically inspired infrastructure.

Finally, the third exhibit delves into the world of vibrations through the exploration of  Chladni plates. By visualizing sound waves as intricate patterns on a vibrating metal plate, visitors gain a deeper understanding of the physics of resonance and wave interference. This exhibit ties the world of acoustics to fundamental principles in physics, offering a visual spectacle of patterns created by vibrating surfaces.

These exhibits aim to captivate audiences by presenting high-level science in interactive and engaging formats, fostering a deeper appreciation for the beauty and complexity of the universe.

Exhibition contents:

1. Ripples of the universe

2. Slime Mold City: Nature’s Blueprint for Bengaluru’s Future Metro Network

3. Chladni plates

1. Ripples of the Universe

In this exhibition, we have tried to mimic binary black hole mergers and subsequently generated Gravitational waves employing a shallow water tank with two rotating balls submerged into the water surface and creating surface waves or ripples on the water surface. Before we dive deep into the system and the analogy between surface waves and Gravitational waves, let us briefly revise a few intriguing concepts as follows.

Introduction to Black Holes and Binary Black Holes

A black hole is a region in space where the gravitational pull is so strong that nothing, not even light, can escape from it. Black holes form when massive stars collapse under their gravity at the end of their life cycles. They are invisible, but their presence can be inferred from the effects they have on nearby objects and light.

A binary black hole system consists of two black holes that are gravitationally bound to each other. They orbit around their common centre of mass, losing energy in the form of gravitational waves (GW), which leads to their eventual merger. 

What Is a Gravitational Wave?

A gravitational wave is like a ripple in the fabric of space and time. Imagine throwing a stone into a pond: the stone creates ripples on the surface of the water that spread out in all directions. Gravitational waves are similar, but instead of water, these ripples travel through space itself!

When Is a Gravitational Wave Generated?

Gravitational waves are generated when massive objects, like black holes or neutron stars, move in a way that creates disturbances in space. For example, if two black holes are spiralling around each other, their intense gravity stirs up space-time around them, creating waves that ripple outward. When these black holes finally collide, the disturbance is so strong that it sends a powerful wave through space.

Space-Time Curvature and Four Dimensions

To understand gravitational waves, it's helpful to think about space and time as being connected in what we call space-time. Imagine space-time as a stretchy, invisible fabric that everything in the universe sits on. Massive objects like planets and stars create dips or curves in this fabric, kind of like how a heavy ball placed on a trampoline makes the surface bend. Now, space-time isn't just a two-dimensional surface; it's four-dimensional. That means it has three dimensions of space (up-down, left-right, forward-backward) and one dimension of time. When something massive moves or changes its position, it doesn't just affect space-it affects time too! These changes in the curvature of space-time can travel as waves, which we detect as gravitational waves.

How Gravitational Waves Affect Us  

These waves are transverse waves which stretch and squeeze the space they move through. But we don’t feel these stretching and squeezing in our everyday lives because the changes of distance made by gravitational waves are incredibly tiny by the time they reach Earth (the typical change in distance caused by a gravitational wave passing through Earth is less than the diameter of a proton (about 10-15 meters)! LIGO's detectors can measure changes in distance as small as one-thousandth of the diameter of a proton, or 10-18 meters.). It takes very sensitive instruments, like the ones used by LIGO (Laser Interferometer Gravitational-Wave Observatory), to detect them.

Frequency of Gravitational Waves

The frequencies of the gravitational waves vary throughout these phases. During the inspiral phase, the frequency increases as the black holes move faster. The merger phase has the highest frequencies, and the ringdown phase features decreasing frequencies as the new black hole stabilizes. These frequencies can range from about 10 Hz to several hundred Hz, with the inspiral phase starting at lower frequencies and the merger phase peaking at higher frequencies.

Why Gravitational Waves Are Important

Gravitational waves are important because they give us a new way to observe the universe. Before we could only study space by looking at light (like with telescopes), but now, with gravitational waves, we can "listen" to the universe as well. This opens up all kinds of exciting possibilities for discovering new things about the cosmos that we couldn't see before!

Binary Black Hole Merging

Binary black hole mergers are among the most fascinating and energetic events in the universe. These events involve two black holes that orbit each other and eventually merge into a single, more massive black hole. The process can be divided into three main phases: the inspiral phase, the merger phase, and the ringdown phase.

1. Inspiral Phase: During this phase, the two black holes spiral closer together due to the emission of gravitational waves. As they lose energy, their orbit shrinks, and they move faster.

2. Merger Phase: This is the moment when the two black holes collide and merge into one. This phase produces the strongest gravitational waves.

3. Ringdown Phase: After the merger, the newly formed black hole settles into a stable state, emitting gravitational waves as it does so.

Why Do Black Holes Merge?

Black holes merge because they emit gravitational waves, which carry away energy and angular momentum from the system. As they lose energy, the black holes spiral closer together until they eventually collide and merge. The process of energy loss through gravitational radiation is like how an orbiting object in space might lose speed and altitude due to friction, although in the case of black holes, it's through the emission of gravitational waves. Watch the following YouTube video for more clarification:

Watch: What if two black holes collided?

Masses of Real Black Holes and Examples of Mergers

Black holes involved in such mergers typically range from a few solar masses to several tens of solar masses. One of the most famous examples is the first direct detection of gravitational waves by LIGO in September 2015. This event, known as GW150914, involved black holes of about 29 and 36 solar masses merging to form a single black hole of approximately 62 solar masses.

How LIGO Detects Gravitational Waves?

LIGO, or the Laser Interferometer Gravitational-wave Observatory, detects gravitational waves by measuring the tiny distortions they cause in space-time. It uses two long arms set at right angles to each other, with laser beams bouncing back and forth. When a gravitational wave passes through, it slightly changes the length of these arms, which is detected by the interference pattern of the lasers.

LIGO India

Analogy Between Shallow Water Waves and Binary Black Hole Mergers

To make these complex phenomena more understandable, scientists often draw analogies. One effective analogy is comparing gravitational waves from binary black hole mergers to shallow water waves in a ripple tank.

Shallow Water Waves as an Analogy

In a ripple tank experiment, two spherical masses rotate in a shallow water tank, creating waves on the water's surface. This setup can mimic the inspiral phase of binary black holes in several ways:

1. Frequency and Amplitude: The amplitude of the GW wave and the shallow water waves dies as 1/R, where R is the distance from the centre of the motor shaft to the region of interest. In the case of GW, the amplitude is proportional to the frequency of the waves. However, in the case of shallow water waves, the amplitude depends on the source pressure exerted on the water. 

2. Wave Propagation: Just as gravitational waves spread out from the black hole merger, water waves propagate outward from the rotating spheres.

3. Sinusoidal Nature: Both gravitational waves and water waves are sinusoidal, meaning they have a smooth, wave-like pattern.

By using a rotating armature to control the frequency of the spheres and a light source to project the wave patterns onto a screen, the ripple tank provides a visual and tangible demonstration of the abstract concept of gravitational waves.

The analogy between shallow-water waves and binary black hole mergers helps to demystify the complex process of gravitational wave generation. By visualizing how rotating masses in water create waves, we can better understand the gravitational waves produced by black holes. This analogy not only aids in scientific education but also sparks curiosity and fascination about the universe among students and the public.

Experimental set-up:

To bring the analogy between shallow-water waves and binary black hole mergers to life, we are using a practical setup involving an Arduino UNO, an L298N motor driver, a DC motor, a power supply, and two 3D-printed balls. Here's how each component contributes to the experiment:

Arduino UNO and L298N Motor Driver

The Arduino UNO serves as the brain of the setup, controlling the speed and movement of the DC motor. The L298N motor driver acts as an interface between the Arduino and the motor, allowing the Arduino to control the motor's direction and speed with precision. This setup lets us adjust the rotation speed of the motor, which directly influences the frequency and amplitude of the waves generated in the water.

DC Motor and 3D-Printed Balls

The DC motor is connected to the motor driver and powered by an external power supply. Hanging from the motor shaft are 3D-printed balls, which are submerged in a shallow water tank, as can be seen in the photo. As the motor rotates, these balls create waves on the water's surface, mimicking the gravitational waves produced by binary black holes during their inspiral phase. By controlling the motor's speed, we can simulate different stages of the black holes' inspiral, with higher speeds representing the final moments before the merger.

Summary

This simple yet effective setup allows us to draw a compelling analogy between shallow-water waves and the gravitational waves generated by binary black holes. By adjusting the motor speed and observing the resulting wave patterns, we can explore and understand the dynamics of one of the most fascinating phenomena in the universe in a hands-on and visual way.

 

Resources: Colliding Supermassive Black Holes, Journey to a Black Hole, The first Binary Black Hole Merger

 

2. Slime Mold City: Nature’s Blueprint for Bengaluru’s Future Metro Network 

At Science Gallery Bengaluru's Sci560 program, we have developed an exciting experiment along with NCBS using the slime mold Physarum polycephalum to model the metro network of Bengaluru. This experiment, on display as part of the exhibit, leverages the natural problem-solving abilities of slime molds to create an efficient and optimized layout for transportation networks. 

Slime molds, despite lacking a brain, have an impressive ability to find the most efficient paths between food sources. In our experiment, we simulate major transit hubs in Bengaluru by placing food (oats) on a map. As the slime mold grows, it extends protoplasmic tubes to connect these food sources, mimicking the way metro lines might link different parts of the city. Over time, the slime mold creates a network that, much like a human-designed metro system, optimizes fault tolerance. 

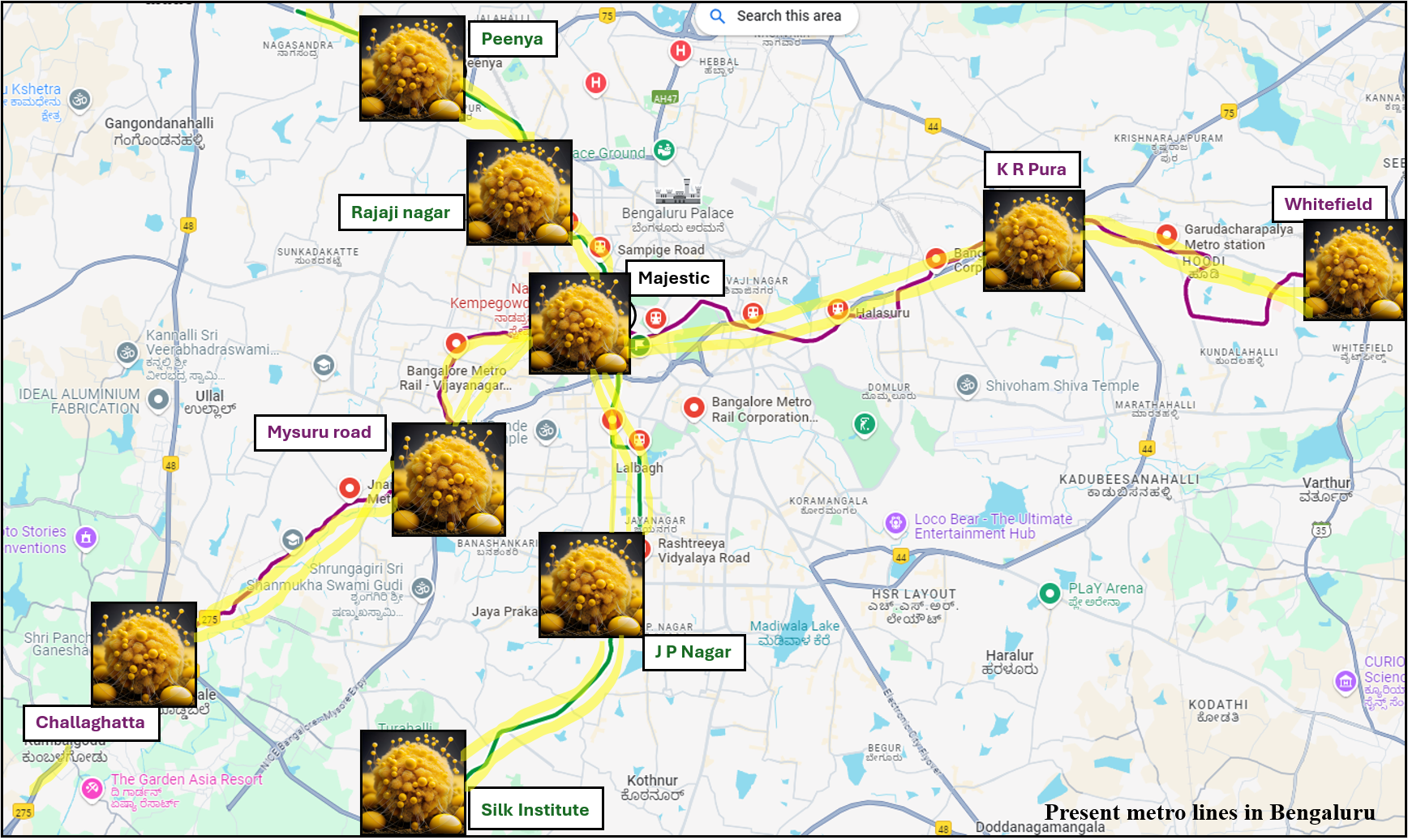
Inspired by the famous Tokyo metro study (A.Tero et al 2010), where Physarum successfully replicated the city’s subway layout, our experiment offers a fascinating glimpse into how nature’s systems can inform human infrastructure. The slime mold’s ability to adapt and reorganize its network (A. Tero et. al. 2010) when faced with environmental changes—such as obstacles or resource scarcity—provides valuable insights into resilient and adaptive network design. These networks and their eventual optimisation are properties that emerge as a result of the contractions and the protoplasmic flows within slime molds. By observing its growth patterns on the Bengaluru map, we can learn how to create more efficient transit systems that minimize redundancy while ensuring robustness.

This exhibit showcases the potential of biologically inspired approaches to urban planning, transportation, and network optimization. It offers a hands-on opportunity for visitors to observe and engage with cutting-edge scientific research that combines biology and technology to solve real-world problems. The images of slime mold growing across the Bengaluru map offers a compelling visual representation of how nature can inspire smarter, more efficient infrastructure design for future cities.

Resources: Emergence, The Blob, Proposed Map

This experiment is done by: Litralson E. R.

 

3. Understanding Chladni Plates: A Journey into the World of Vibrations

What is a Chladni Plate?

A Chladni plate is a thin, usually metal, plate that vibrates when excited by sound waves or mechanical forces. These vibrations cause the plate to resonate at certain frequencies, producing beautiful and intricate patterns that can be seen by sprinkling fine powder (like sand or salt) on its surface. These patterns form because the powder accumulates along the nodal lines, where the plate does not move, creating a visual representation of the vibrational modes.

The Chladni plate was discovered by Ernst Chladni, a German physicist and musician, in the late 18th century. Chladni was interested in the study of sound and vibration, which led him to explore how different materials respond to vibrations. He was particularly fascinated by the idea of making sound waves visible, allowing people to "see" the effects of sound on a material surface. This discovery was a breakthrough in acoustics and laid the foundation for modern studies in vibration physics, instrument design, and even engineering.

Chladni's work helped answer fundamental questions about how different parts of a surface vibrate, which was essential for understanding the physics of sound and for improving the design of musical instruments. By visualizing the vibration patterns, Chladni could explain why different shapes and materials produce different sounds, leading to better-tuned instruments.

Drawing an Analogy: Vibrating String vs. Chladni Plate Vibrations

To understand Chladni plate vibrations, it's helpful to draw an analogy to something simpler and more familiar: a vibrating string, like the ones on a guitar or a violin.

Vibrating String: When you pluck a string, it vibrates back and forth, creating waves that travel along the length of the string. These waves reflect off the ends of the string, interfering with each other and creating standing waves. The points where the string doesn’t move are called nodes, and the points where the string vibrates the most are called antinodes. These vibrations produce sound, and the frequency of the vibration determines the pitch.

Chladni Plate: The Chladni plate behaves similarly, but instead of a one-dimensional string, we have a two-dimensional surface. When the plate is vibrated at certain frequencies, it forms standing waves across its surface. Just like with the string, there are nodes (where the plate doesn’t move) and antinodes (where the plate vibrates the most). The difference is that these vibrations create complex, two-dimensional patterns rather than a single, simple wave. The powder on the plate collects at the nodes, making the vibration patterns visible.

So, both the vibrating string and the Chladni plate are examples of standing waves, but the Chladni plate offers a richer and more complex set of patterns because it’s a two-dimensional surface.

Nodes and Antinodes in Chladni Plate Patterns

When a Chladni plate vibrates, it forms regions where the surface moves a lot (antinodes) and regions where the surface doesn’t move at all (nodes). The powder sprinkled on the plate settles in the nodal lines because those are the places where there is no movement. This creates a visible pattern that corresponds to the vibrational mode of the plate.

Nodes: These are the points or lines on the plate where there is no movement during vibration. The powder collects here because these regions are stationary.

Antinodes: These are the areas of maximum movement, where the plate vibrates the most. The powder is pushed away from these regions.

The patterns you see on the plate are the result of how these nodes and antinodes distribute themselves across the surface. As the frequency of vibration changes, the positions of nodes and antinodes shift, leading to different patterns.

Why Do These Patterns Form?

The patterns form because of the way sound waves interfere with each other on the surface of the plate. When the frequency is just right, the waves constructively and destructively interfere to create standing waves, which lead to the formation of nodes and antinodes. These standing waves are essentially the plate’s way of "resonating" at a particular frequency, and each pattern corresponds to a different mode of vibration. The patterns can be found in the image and videos below:

Please watch the following videos to get more ideas about the exhibit

Watch: Chladni plate vibrating with a Vibration generator

Watch: Chladni plate vibrating with a Violin bow

How Do the Size and Shape of the Metal Plate Affect Pattern Formations?

The size and shape of a metal plate significantly impact the patterns of nodes and antinodes that form during vibration. When the size of a plate is doubled, for instance, the wavelengths of the standing waves that can fit across the plate also change. Specifically, as the size of a square plate is increased, the frequencies required to produce the same vibrational modes decrease, and the patterns become more intricate, with more nodes and antinodes appearing on the surface. This is because a larger plate can support longer wavelengths, allowing for more complex interference patterns.

Changing the shape of the plate has an even more profound effect on the patterns formed. A square plate, due to its symmetrical geometry, tends to produce symmetric and regular nodal patterns, often with straight lines or simple curves dividing the plate into equal sections. If the plate's shape is changed to a circle, the symmetry of the plate is altered, resulting in circular or radial nodal patterns. The modes generated in a circular plate often feature concentric rings or radial lines as nodes, which are quite different from the straight or grid-like patterns seen in square plates. The change in geometry alters the boundary conditions for the waves, leading to a different set of natural frequencies and thus a distinct set of vibrational modes. Therefore, both the size and shape of the plate are crucial factors in determining the appearance and complexity of the nodal patterns that emerge.

Harmonic and Anharmonic Modes on a Square-Shaped Metal Chladni Plate

When we talk about the "modes" of vibration on a Chladni plate, we’re referring to the specific patterns of nodes and antinodes that form at different frequencies. On a square-shaped plate, these modes can be either harmonic or anharmonic.

Harmonic Modes: These are the simplest and most symmetric patterns. For a square plate, a harmonic mode might have nodes that are straight lines dividing the plate into equal sections, like a checkerboard. The frequency that produces a harmonic mode is usually related to the fundamental frequency, which is the lowest frequency at which the plate can vibrate.

Anharmonic Modes: These modes are more complex and irregular. They occur at frequencies that are not simple multiples of the fundamental frequency. The patterns can be asymmetric, with nodes forming curved lines or more complicated shapes. These modes are harder to predict and can vary significantly depending on the exact shape and material of the plate.

How Can We Know the Different Modes?

To identify the different modes on a square-shaped Chladni plate, you can:

1. Experiment with Different Frequencies: By gradually increasing the frequency of vibration, you can observe how the patterns change. Each new pattern corresponds to a different vibrational mode.

2. Use Mathematical Models: Physicists use mathematical equations to predict the frequencies at which different modes will occur. For a square plate, these predictions take into account the plate’s dimensions, material properties, and boundary conditions (how the plate is held or clamped).

3. Visual Inspection: Simply observing the patterns as you vary the frequency can give you an idea of the harmonic and anharmonic modes. For instance, a simple pattern with straight lines is likely a harmonic mode, while a more intricate pattern is likely anharmonic.

Conclusion

Chladni plates are a wonderful demonstration of the principles of vibration and wave interference. They show us how sound waves can create beautiful, intricate patterns and help us understand the underlying physics of resonance and standing waves. Whether you’re a science enthusiast or a curious learner, studying Chladni plates offers a fascinating glimpse into the world of acoustics and vibration physics.

Resources: Chladni plates, How Chladni plates make it possible to visualise sound waves

Credits:

Abdul Hakkim

Akshit Goyal

Ajith P.

Disha K.

Ikbal Ahmed

Joseph Samuel

Litralson E. R. (NCBS)

Outreach ICTS

Prayush Kumar

Shashi Thutupalli (ICTS & NCBS)

Vishal Vasan

 

Images and videos are sourced from: https://nervoussquirrel.com/chladni_plates.html, Adobe Firefly/ICTS, Summer School for Women in Physics 2023, ICTS Labs, Science Gallery Bengaluru, YouTube.

Contact us: outreach@icts.res.in and/or ikbal.ahmed@icts.res.in