Monday, 11 September 2023
In this mini-course, we shall give a basic introduction to the topics listed in the title. In the first lecture, we shall introduce the main players and state the modularity theorem for elliptic curves. In the second lecture, we shall introduce Hecke operators on modular forms and modular curves. In the third lecture, we shall introduce the Jacobians of modular curves. In the last lecture, we shall describe how integral models of modular curves can be used in conjunction with the $q$-expansion principle to give an integral structure to the space of modular forms.
References:
1. Diamond, Fred; Shurman, Jerry. A first course in modular forms. Graduate Texts in Mathematics, 228. Springer-Verlag, New York, 2005.
2. Diamond, Fred; Im, John. Modular forms and modular curves. Seminar on Fermat's Last Theorem (Toronto, ON, 1993–1994), 39--133, CMS Conf. Proc., 17, Amer. Math. Soc., Providence, RI, 1995.
In this course, we will give a general overview of the Chabauty method(s), going from the classical (linear) Chabauty to quadratic Chabauty (with maybe a bit of "geometric quadratic Chabauty" at the end), explaining whenever possible how to turn the theoretical results into explicit and somewhat down-to-earth computations.
In this course, we will study the arithmetic properties of special points on modular curves. The main focus will be on rational points on modular Jacobians arising from the theory of complex multiplication. After a brief discussion of the basics of the theory, we give an overview of some of the most important results of the latter half of the 20th century concerning the question of when such points are non-torsion. Some of the main ideas of two results in particular will be our primary focus: Mazur’s work on torsion on elliptic curves, and the work of Gross-Zagier on heights of Heegner points.
References:
[DI95] Diamond, Fred; Im, John. Modular forms and modular curves. Seminar on Fermat’s Last Theorem (Toronto, ON, 1993–1994), 39–133, CMS Conf. Proc., 17, Amer. Math. Soc., Providence, RI, 1995.
[Gro85] Gross, Benedict H.; Zagier, Don B. On singular moduli. J. Reine Angew. Math. 355 (1985), 191–220.
[Gro87] Gross, B.; Kohnen, W.; Zagier, D. Heegner points and derivatives of L-series. II. Math. Ann. 278 (1987), no. 1-4, 497–562.
[Kol91] Kolyvagin, V. A. On the structure of Shafarevich-Tate groups. Algebraic geometry (Chicago, IL, 1989), 94–121, Lecture Notes in Math., 1479, Springer, Berlin, 1991.
[Lan87] Lang, Serge. Elliptic functions. With an appendix by J. Tate. Second edition. Graduate Texts in Mathematics, 112. Springer-Verlag, New York, 1987.
[Maz78] Mazur, B. Modular curves and the Eisenstein ideal. With an appendix by Mazur and M. Rapoport. Inst. Hautes Etudes Sci. Publ. Math. No. 47 (1977), 33–186 (1978). ́
[MazD78] Mazur, B. Rational isogenies of prime degree (with an appendix by D. Goldfeld). Invent. Math. 44 (1978), no. 2, 129–162.
[Wei76] Weil, Andr ́e. Elliptic functions according to Eisenstein and Kronecker. Reprint of the 1976 original. Classics in Mathematics. Springer-Verlag, Berlin, 1999.
Though the instructor will recall some of the necessary material during his course, the familiarity with the following topics may be useful.
• The basic knowledge on Elliptic Curves (Silverman’s book on Arithmetic of Elliptic curves), including the method of descent, and the theory of complex multiplication.
• The basic theory of modular curves as algebraic moduli spaces, the basic theory of modular forms, Wiles’ modularity theorem.


Tuesday, 12 September 2023
In this course, we will give a general overview of the Chabauty method(s), going from the classical (linear) Chabauty to quadratic Chabauty (with maybe a bit of "geometric quadratic Chabauty" at the end), explaining whenever possible
how to turn the theoretical results into explicit and somewhat down-to-earth computations.
In this mini-course, we shall give a basic introduction to the topics listed in the title. In the first lecture, we shall introduce the main players and state the modularity theorem for elliptic curves. In the second lecture, we shall introduce Hecke operators on modular forms and modular curves. In the third lecture, we shall introduce the Jacobians of modular curves. In the last lecture, we shall describe how integral models of modular curves can be used in conjunction with the $q$-expansion principle to give an integral structure to the space of modular forms.
References:
1. Diamond, Fred; Shurman, Jerry. A first course in modular forms. Graduate Texts in Mathematics, 228. Springer-Verlag, New York, 2005.
2. Diamond, Fred; Im, John. Modular forms and modular curves. Seminar on Fermat's Last Theorem (Toronto, ON, 1993–1994), 39--133, CMS Conf. Proc., 17, Amer. Math. Soc., Providence, RI, 1995.
In this course, we will study the arithmetic properties of special points on modular curves. The main focus will be on rational points on modular Jacobians arising from the theory of complex multiplication. After a brief discussion of the basics of the theory, we give an overview of some of the most important results of the latter half of the 20th century concerning the question of when such points are non-torsion. Some of the main ideas of two results in particular will be our primary focus: Mazur’s work on torsion on elliptic curves, and the work of Gross-Zagier on heights of Heegner points.
References:
[DI95] Diamond, Fred; Im, John. Modular forms and modular curves. Seminar on Fermat’s Last Theorem (Toronto, ON, 1993–1994), 39–133, CMS Conf. Proc., 17, Amer. Math. Soc., Providence, RI, 1995.
[Gro85] Gross, Benedict H.; Zagier, Don B. On singular moduli. J. Reine Angew. Math. 355 (1985), 191–220.
[Gro87] Gross, B.; Kohnen, W.; Zagier, D. Heegner points and derivatives of L-series. II. Math. Ann. 278 (1987), no. 1-4, 497–562.
[Kol91] Kolyvagin, V. A. On the structure of Shafarevich-Tate groups. Algebraic geometry (Chicago, IL, 1989), 94–121, Lecture Notes in Math., 1479, Springer, Berlin, 1991.
[Lan87] Lang, Serge. Elliptic functions. With an appendix by J. Tate. Second edition. Graduate Texts in Mathematics, 112. Springer-Verlag, New York, 1987.
[Maz78] Mazur, B. Modular curves and the Eisenstein ideal. With an appendix by Mazur and M. Rapoport. Inst. Hautes Etudes Sci. Publ. Math. No. 47 (1977), 33–186 (1978). ́
[MazD78] Mazur, B. Rational isogenies of prime degree (with an appendix by D. Goldfeld). Invent. Math. 44 (1978), no. 2, 129–162.
[Wei76] Weil, Andr ́e. Elliptic functions according to Eisenstein and Kronecker. Reprint of the 1976 original. Classics in Mathematics. Springer-Verlag, Berlin, 1999.
Though the instructor will recall some of the necessary material during his course, the familiarity with the following topics may be useful.
• The basic knowledge on Elliptic Curves (Silverman’s book on Arithmetic of Elliptic curves), including the method of descent, and the theory of complex multiplication.
• The basic theory of modular curves as algebraic moduli spaces, the basic theory of modular forms, Wiles’ modularity theorem.
We give an overview of the computational tools that are useful for studying various aspects of the Chabauty--Coleman and nonabelian Chabauty methods. We will illustrate carrying out the
method on various modular curves.
References
[BM20] Balakrishnan, Jennifer; M ̈uller, Steffen (2020). Arizona Winter School Lectures. http://math.bu.edu/people/jbala/2020BalakrishnanMuellerNotes.pdf
Wednesday, 13 September 2023
In this course, we will study the arithmetic properties of special points on modular curves. The main focus will be on rational points on modular Jacobians arising from the theory of complex multiplication. After a brief discussion of the basics of the theory, we give an overview of some of the most important results of the latter half of the 20th century concerning the question of when such points are non-torsion. Some of the main ideas of two results in particular will be our primary focus: Mazur’s work on torsion on elliptic curves, and the work of Gross-Zagier on heights of Heegner points.
References:
[DI95] Diamond, Fred; Im, John. Modular forms and modular curves. Seminar on Fermat’s Last Theorem (Toronto, ON, 1993–1994), 39–133, CMS Conf. Proc., 17, Amer. Math. Soc., Providence, RI, 1995.
[Gro85] Gross, Benedict H.; Zagier, Don B. On singular moduli. J. Reine Angew. Math. 355 (1985), 191–220.
[Gro87] Gross, B.; Kohnen, W.; Zagier, D. Heegner points and derivatives of L-series. II. Math. Ann. 278 (1987), no. 1-4, 497–562.
[Kol91] Kolyvagin, V. A. On the structure of Shafarevich-Tate groups. Algebraic geometry (Chicago, IL, 1989), 94–121, Lecture Notes in Math., 1479, Springer, Berlin, 1991.
[Lan87] Lang, Serge. Elliptic functions. With an appendix by J. Tate. Second edition. Graduate Texts in Mathematics, 112. Springer-Verlag, New York, 1987.
[Maz78] Mazur, B. Modular curves and the Eisenstein ideal. With an appendix by Mazur and M. Rapoport. Inst. Hautes Etudes Sci. Publ. Math. No. 47 (1977), 33–186 (1978). ́
[MazD78] Mazur, B. Rational isogenies of prime degree (with an appendix by D. Goldfeld). Invent. Math. 44 (1978), no. 2, 129–162.
[Wei76] Weil, Andr ́e. Elliptic functions according to Eisenstein and Kronecker. Reprint of the 1976 original. Classics in Mathematics. Springer-Verlag, Berlin, 1999.
Though the instructor will recall some of the necessary material during his course, the familiarity with the following topics may be useful.
• The basic knowledge on Elliptic Curves (Silverman’s book on Arithmetic of Elliptic curves), including the method of descent, and the theory of complex multiplication.
• The basic theory of modular curves as algebraic moduli spaces, the basic theory of modular forms, Wiles’ modularity theorem.
In the first part of this course I will discuss unipotent flat connections and unipotent isocrystals on varieties, from a theoretical and computational perspective. This will lead to a notion of iterated p-adic integrals as introduced by Coleman and Besser. In the second part, I will describe the Chabauty–Coleman–Kim method can sometimes be used to prove that these p-adic integrals satisfy ‘special’ identities when evaluated on rational points. I will also give some examples of how this can lead to a method for determining the set of rational points.
References:
[Ber] Berthelot, Pierre. Cohomologie rigide et cohomologie rigide `a support propre, `aparaˆıtre dans Ast ́erisque.
[Bes12] Besser, Amnon. Heidelberg lectures on Coleman integration. The arithmetic of fundamental groups–PIA 2010, 3–52, Contrib. Math. Comput. Sci., 2, Springer, Heidelberg, 2012.
[Bes02] Besser, Amnon. Coleman integration using the Tannakian formalism. Math. Ann. 322 (2002), no. 1, 19–48.
[Ked01] Kedlaya, Kiran S. Counting points on hyperelliptic curves using Monsky-Washnitzer cohomology. J. Ramanujan Math. Soc. 16 (2001), no. 4, 323–338.
[Kim05] Kim, Minhyong. The motivic fundamental group of P 1 \ {0, 1, ∞} and the theorem of Siegel. Invent. Math. 161 (2005), no. 3, 629–656.
[Kim09] Kim, Minhyong. The unipotent Albanese map and Selmer varieties for curves. Publ. Res. Inst. Math. Sci. 45 (2009), no. 1, 89–133.
In this mini-course, we shall give a basic introduction to the topics listed in the title. In the first lecture, we shall introduce the main players and state the modularity theorem for elliptic curves. In the second lecture, we shall introduce Hecke operators on modular forms and modular curves. In the third lecture, we shall introduce the Jacobians of modular curves. In the last lecture, we shall describe how integral models of modular curves can be used in conjunction with the $q$-expansion principle to give an integral structure to the space of modular forms.
References:
1. Diamond, Fred; Shurman, Jerry. A first course in modular forms. Graduate Texts in Mathematics, 228. Springer-Verlag, New York, 2005.
2. Diamond, Fred; Im, John. Modular forms and modular curves. Seminar on Fermat's Last Theorem (Toronto, ON, 1993–1994), 39--133, CMS Conf. Proc., 17, Amer. Math. Soc., Providence, RI, 1995.
We give an overview of the computational tools that are useful for studying various aspects of the Chabauty--Coleman and nonabelian Chabauty methods. We will illustrate carrying out the method on various modular curves.
Thursday, 14 September 2023
In the first part of this course I will discuss unipotent flat connections and unipotent isocrystals on varieties, from a theoretical and computational perspective. This will lead to a notion of iterated p-adic integrals as introduced by Coleman and Besser. In the second part, I will describe the Chabauty–Coleman–Kim method can sometimes be used to prove that these p-adic integrals satisfy ‘special’ identities when evaluated on rational points. I will also give some examples of how this can lead to a method for determining the set of rational points.
References:
[Ber] Berthelot, Pierre. Cohomologie rigide et cohomologie rigide `a support propre, `aparaˆıtre dans Ast ́erisque.
[Bes12] Besser, Amnon. Heidelberg lectures on Coleman integration. The arithmetic of fundamental groups–PIA 2010, 3–52, Contrib. Math. Comput. Sci., 2, Springer, Heidelberg, 2012.
[Bes02] Besser, Amnon. Coleman integration using the Tannakian formalism. Math. Ann. 322 (2002), no. 1, 19–48.
[Ked01] Kedlaya, Kiran S. Counting points on hyperelliptic curves using Monsky-Washnitzer cohomology. J. Ramanujan Math. Soc. 16 (2001), no. 4, 323–338.
[Kim05] Kim, Minhyong. The motivic fundamental group of P 1 \ {0, 1, ∞} and the theorem of Siegel. Invent. Math. 161 (2005), no. 3, 629–656.
[Kim09] Kim, Minhyong. The unipotent Albanese map and Selmer varieties for curves. Publ. Res. Inst. Math. Sci. 45 (2009), no. 1, 89–133.
In this course, we will study the arithmetic properties of special points on modular curves. The main focus will be on rational points on modular Jacobians arising from the theory of complex multiplication. After a brief discussion of the basics of the theory, we give an overview of some of the most important results of the latter half of the 20th century concerning the question of when such points are non-torsion. Some of the main ideas of two results in particular will be our primary focus: Mazur’s work on torsion on elliptic curves, and the work of Gross-Zagier on heights of Heegner points.
References:
[DI95] Diamond, Fred; Im, John. Modular forms and modular curves. Seminar on Fermat’s Last Theorem (Toronto, ON, 1993–1994), 39–133, CMS Conf. Proc., 17, Amer. Math. Soc., Providence, RI, 1995.
[Gro85] Gross, Benedict H.; Zagier, Don B. On singular moduli. J. Reine Angew. Math. 355 (1985), 191–220.
[Gro87] Gross, B.; Kohnen, W.; Zagier, D. Heegner points and derivatives of L-series. II. Math. Ann. 278 (1987), no. 1-4, 497–562.
[Kol91] Kolyvagin, V. A. On the structure of Shafarevich-Tate groups. Algebraic geometry (Chicago, IL, 1989), 94–121, Lecture Notes in Math., 1479, Springer, Berlin, 1991.
[Lan87] Lang, Serge. Elliptic functions. With an appendix by J. Tate. Second edition. Graduate Texts in Mathematics, 112. Springer-Verlag, New York, 1987.
[Maz78] Mazur, B. Modular curves and the Eisenstein ideal. With an appendix by Mazur and M. Rapoport. Inst. Hautes Etudes Sci. Publ. Math. No. 47 (1977), 33–186 (1978). ́
[MazD78] Mazur, B. Rational isogenies of prime degree (with an appendix by D. Goldfeld). Invent. Math. 44 (1978), no. 2, 129–162.
[Wei76] Weil, Andr ́e. Elliptic functions according to Eisenstein and Kronecker. Reprint of the 1976 original. Classics in Mathematics. Springer-Verlag, Berlin, 1999.
Though the instructor will recall some of the necessary material during his course, the familiarity with the following topics may be useful.
• The basic knowledge on Elliptic Curves (Silverman’s book on Arithmetic of Elliptic curves), including the method of descent, and the theory of complex multiplication.
• The basic theory of modular curves as algebraic moduli spaces, the basic theory of modular forms, Wiles’ modularity theorem.
In this course, we will give a general overview of the Chabauty method(s), going from the classical (linear) Chabauty to quadratic Chabauty (with maybe a bit of "geometric quadratic Chabauty" at the end), explaining whenever possible how to turn the theoretical results into explicit and somewhat down-to-earth computations.
We give an overview of the computational tools that are useful for studying various aspects of the Chabauty--Coleman and nonabelian Chabauty methods. We will illustrate carrying out the method on various modular curves.
Friday, 15 September 2023
In the first part of this course I will discuss unipotent flat connections and unipotent isocrystals on varieties, from a theoretical and computational perspective. This will lead to a notion of iterated p-adic integrals as introduced by Coleman and Besser. In the second part, I will describe the Chabauty–Coleman–Kim method can sometimes be used to prove that these p-adic integrals satisfy ‘special’ identities when evaluated on rational points. I will also give some examples of how this can lead to a method for determining the set of rational points.
References:
[Ber] Berthelot, Pierre. Cohomologie rigide et cohomologie rigide `a support propre, `aparaˆıtre dans Ast ́erisque.
[Bes12] Besser, Amnon. Heidelberg lectures on Coleman integration. The arithmetic of fundamental groups–PIA 2010, 3–52, Contrib. Math. Comput. Sci., 2, Springer, Heidelberg, 2012.
[Bes02] Besser, Amnon. Coleman integration using the Tannakian formalism. Math. Ann. 322 (2002), no. 1, 19–48.
[Ked01] Kedlaya, Kiran S. Counting points on hyperelliptic curves using Monsky-Washnitzer cohomology. J. Ramanujan Math. Soc. 16 (2001), no. 4, 323–338.
[Kim05] Kim, Minhyong. The motivic fundamental group of P 1 \ {0, 1, ∞} and the theorem of Siegel. Invent. Math. 161 (2005), no. 3, 629–656.
[Kim09] Kim, Minhyong. The unipotent Albanese map and Selmer varieties for curves. Publ. Res. Inst. Math. Sci. 45 (2009), no. 1, 89–133.
In this course, we will give a general overview of the Chabauty method(s), going from the classical (linear) Chabauty to quadratic Chabauty (with maybe a bit of "geometric quadratic Chabauty" at the end), explaining whenever possible how to turn the theoretical results into explicit and somewhat down-to-earth computations.
In this mini-course, we shall give a basic introduction to the topics listed in the title. In the first lecture, we shall introduce the main players and state the modularity theorem for elliptic curves. In the second lecture, we shall introduce Hecke operators on modular forms and modular curves. In the third lecture, we shall introduce the Jacobians of modular curves. In the last lecture, we shall describe how integral models of modular curves can be used in conjunction with the $q$-expansion principle to give an integral structure to the space of modular forms.
1. Diamond, Fred; Shurman, Jerry. A first course in modular forms. Graduate Texts in Mathematics, 228. Springer-Verlag, New York, 2005.
2. Diamond, Fred; Im, John. Modular forms and modular curves. Seminar on Fermat's Last Theorem (Toronto, ON, 1993–1994), 39--133, CMS Conf. Proc., 17, Amer. Math. Soc., Providence, RI, 1995.
In the first part of this course I will discuss unipotent flat connections and unipotent isocrystals on varieties, from a theoretical and computational perspective. This will lead to a notion of iterated p-adic integrals as introduced by Coleman and Besser. In the second part, I will describe the Chabauty–Coleman–Kim method can sometimes be used to prove that these p-adic integrals satisfy ‘special’ identities when evaluated on rational points. I will also give some examples of how this can lead to a method for determining the set of rational points.
References:
[Ber] Berthelot, Pierre. Cohomologie rigide et cohomologie rigide `a support propre, `aparaˆıtre dans Ast ́erisque.
[Bes12] Besser, Amnon. Heidelberg lectures on Coleman integration. The arithmetic of fundamental groups–PIA 2010, 3–52, Contrib. Math. Comput. Sci., 2, Springer, Heidelberg, 2012.
[Bes02] Besser, Amnon. Coleman integration using the Tannakian formalism. Math. Ann. 322 (2002), no. 1, 19–48.
[Ked01] Kedlaya, Kiran S. Counting points on hyperelliptic curves using Monsky-Washnitzer cohomology. J. Ramanujan Math. Soc. 16 (2001), no. 4, 323–338.
[Kim05] Kim, Minhyong. The motivic fundamental group of P 1 \ {0, 1, ∞} and the theorem of Siegel. Invent. Math. 161 (2005), no. 3, 629–656.
[Kim09] Kim, Minhyong. The unipotent Albanese map and Selmer varieties for curves. Publ. Res. Inst. Math. Sci. 45 (2009), no. 1, 89–133.
Monday, 18 September 2023

In this talk I will discuss a joint work with Netan Dogra, showing that by analytic tools and a slight extension of quadratic Chabauty condition that this method applies in principle to all nonsplit Cartan modular curves (of genus at least 1) to provide at least a bound on their number of rational points
Recently, Caro and Pasten have generalised Coleman's bound on the number of rational points on curves of low Mordell--Weil rank to certain surfaces in abelian varieties of Mordell--Weil rank 1. In this talk I will describe a result that generalises their result to higher dimension and larger Mordell--Weil ranks.
Modular varieties associated with unitary and orthogonal groups contain codimension one subvarieties of the same type -- in the classical case these would be Heegner points on modular curves. The injectivity of restriction of cohomology to such divisors is referred to as an automorphic Lefschetz property; the question is to decide when this holds and in what degrees. I will describe joint work with collaborators in which we study the question for some noncompact modular varieties, generalizing results by several authors, and also try to place the question in the correct framework, that of branching laws and L-values.

Tuesday, 19 September 2023
Ribet’s method, and its refinements by Wiles, is the only systematic method of producing non-trivial elements in Selmer groups. If chi_1 and chi_2 are two characters from a compact group into a complete local northerian local ring R, then Ribet’s lemma gives a strategy of constructing an extension of chi_1 by chi_2. An important assumption that occurs in Ribet’s lemma is that chi_1 and chi_2 are distinct modulo the maximal ideal of R. In this talk I will give a formulation of Ribet’s Lemma without this assumption and sketch its proof. This is joint work with Samit Dasgupta, Jesse Silliman and Jiuya Wang.
This talk is about the problem of counting rational points of bounded height on (stacky) modular curves whose coarse moduli space has genus 0. First, we will survey various older and newer definitions of height functions on stacks, with a focus on weighted projective stacks. Next, we will give an overview of recent results on counting points with respect to such height functions. Finally, we will give applications to counting elliptic curves with prescribed level structure over number fields. This is partly based on joint work with Filip Najman and with Irati Manterola Ayala.
In recent years, the analogy between knots and primes has been recast in the language of topological quantum field theory. This talk will give a review of these developments with a particular focus on elliptic curves.
Wednesday, 20 September 2023
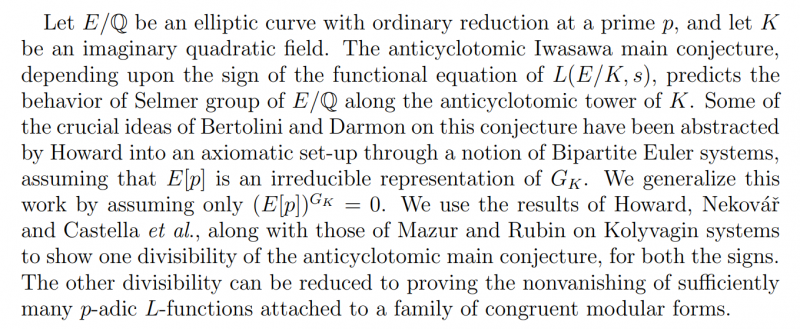
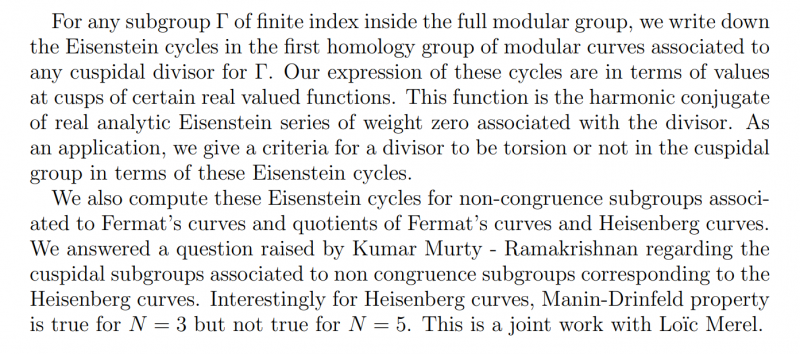
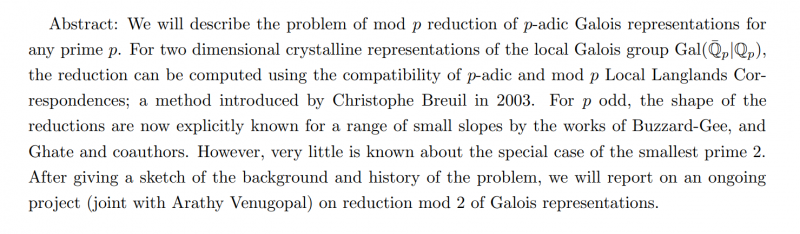
p-adic heights have been a rich source of explicit p-adic analytic functions vanishing on rational points on a curve. In this talk, we will outline a new construction of canonical p-adic heights for line bundles on abelian varieties from p-adic adelic metrics. This uses p-adic Arakelov theory developed by Besser, and is a non-Archimedean analogue of Zhang's construction of canonical real valued heights from real-valued adelic metrics. We will use this new construction to give direct explanations (avoiding p-adic Hodge theory) of the key properties of height pairings needed for the quadratic Chabauty method for finding rational points. This is joint work with Amnon Besser and Steffen Mueller.
The Chabauty–Kim program for studying rational points on hyperbolic curves yields a series of refined Selmer sets at depth n, cut out by n-fold iterated p-adic integrals. We discuss a collection of examples of computations that have been done of these sets in depth 1 and 2, in cases including punctured elliptic curves and smooth projective curves of genus 2 and 3.
Thursday, 21 September 2023

We will discuss equidistribution of matrix valued Gauss sums, that are associated to general and self-dual cuspidal representations of the general linear group over finite fields. These are also related to local root numbers. This is joint work with Sameer Kulkarni.
Special values of modular functions at points in imaginary quadratic fields have traditionally played an important role in arithmetic, and their algebraic nature is the source of systematic constructions of rational points on modular curves. In this talk, I will discuss recent progress in establishing notions of singular moduli for real quadratic fields, through a study of certain p-adic analytic families of automorphic forms.
Quadratic Chabauty (QC) is the first nontrivial part of the Chabauty-Kim (CK) method and has had a lot of success in provably finding sets of rational points on hyperbolic curves, for example solving some outstanding questions in the theory of modular curves. Nonetheless, QC does not apply to all curves, and while CK is theoretically expected to work for all hyperbolic curves, very little has been done explicitly - and almost entirely in the genus 0 case. We discuss a variety of works in progress, some joint with Ishai Dan-Cohen, to make CK explicit and computational beyond the quadratic and genus 0 cases.
Let A and A′ be abelian varieties defined over a number field K. In the talk I will consider the following question: Is it true that A and A′ are quadratic twists of one another if and only if they are quadratic twists modulo p for almost every primes p of K? Serre and Ramakrishnan have given a positive answer in the case of elliptic curves and a result of Rajan implies the validity of the principle when the endomorphism ring of A (and hence also that of A′ ) over an algebraic closure of ℚ is just Z. For not necessarily simple abelian varieties, I will show that the answer is affirmative up to dimension 3, but that it becomes negative in dimension 4. Time permitting, I will present ongoing joint work with E. Ambrosi that leads to more general results.
Friday, 22 September 2023
This talk will present an overview of the results known for the fine Selmer group over anticyclotomic extensions, in particular the Iwasawa invariants. We also present the results for the different Selmer groups over such extensions.
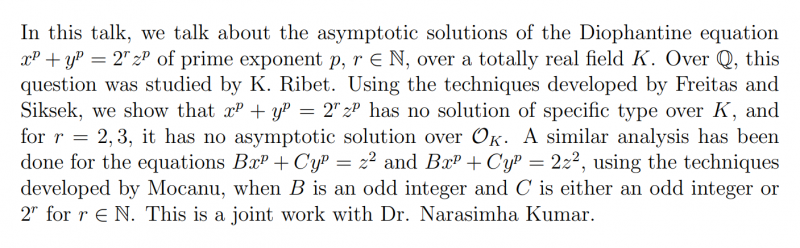
I will explain a new approach to generalized Fermat equations of signature (r,r,p) based on multi-Frey techniques and ideas from Darmon’s program.
In 1974, Arakelov introduced an intersection theory on arithmetic surfaces. By its very definition, it is the sum of a geometric part that encodes the finite intersection of divisors coming from the cusps, and an analytic part which is given in terms of the canonical Green’s function at cusps. I will talk about the Arakelov self-intersection number of the relative dualizing sheaf of Edixhovens minimal regular model for certain modular curves.